An Investigation of Seismic Behaviour of Prestressed Concrete Frame Structures using Pushover Analysis

Abstract

The prestressed concrete system is a prominent material in civil engineering, with applications ranging from buildings, bridges, foundations, piling, silos, stadiums, roadways, and other infrastructure. However, this work is primarily concerned with its applicability to building frame constructions subjected to seismic loading. The prestressed concrete system for frame constructions not only reduces the amount of structural components, but it is also a cost-effective technology that provides outstanding strength and stiffness, as well as quick and simple site erection. Hence, we will be able to use these prestressed concrete components to their full capacity, unlocking engineering and social benefits that were previously impossible. Prestressed concrete designs, as opposed to reinforced concrete designs, can give structures with substantially longer spans, no cracks, or fewer cracks with tiny crack widths. Nonetheless, research on the seismic behaviour of prestressed concrete frame structures is relatively restricted, with the majority of studies focusing on reinforced concrete structures. Finally, the provisional versions of modern building codes such as ACI 318, GB50010, and EC2 do not provide thorough processes for seismic design of prestressed concrete structures. In this work, the seismic design and behaviour of prestressed concrete building frames are explored using the most recent design code. Three model-building codes will be investigated and compared, including ACI 318-14, Eurocode 2004-2, and Chinese GB500010-2010. The 10-story prestressed concrete frame system is subjected to gravity and seismic loading, and the strong-column weak beam mechanism is archived to conform with the newly implemented building design code. To examine the behaviour of prestressed concrete frame structures, nonlinear static pushover analysis is used to capture the reaction under earthquake loading. The stiffness and strength needed will be designed in compliance with the three major building codes indicated. In addition, the finite element model will be created using CSI Sap200 to capture its actions.

Introduction

I. INTRODUCTION

Historically, seismic design has been based on the single criterion of ensuring life safety during a design level earthquake. This goal has been found to be most economically accomplished by allowing the structure to respond in-elastically to earthquake loading, which limits the maximum force that any specific structural element will encounter while also lowering the peak response of the structures due to hysteretic energy dissipation. However, due to this design philosophy, most structures are expected to incur deformation after a moderate to severe earthquake; this damage may include structural system stiffness and strength loss, as well as lingering deformations. This may result in costly structural repairs and unfavourable restrictions on building use while those maintenances are performed. Structural engineers are increasingly recognizing that life safety is an important but not always sufficient performance goal. It would be wonderful if a structure could be built cheaply to assure life safety while also remaining uninjured or substantially undamaged in the case of a major earthquake.

II. LITERATURE REVIEW

An investigation of the use of prestressed concrete structures under seismic loads has been carried out by Nakano, Sutherland, and Lin. The findings show that structures made of prestressed concrete are sufficiently resilient to withstand mild to severe seismic excitation[1][2][3]. Inomata examined the behaviour of the twelve reinforced concrete and prestressed concrete beam samples under the scenario of cyclic loading[4]. To increase ductility and lessen the excessive loss of stiffness and strength, the researcher recommended that prestressed concrete members be constructed in compliance with FIP-CEB guidelines. When developed utilizing the recommended method, the prestressed member has significant ductility in comparison to the standard reinforced concrete part.

Hawkins examines the phenomenon of analysis and experimental outcomes of twenty-eight sub-assemblages of precast and prestressed concrete joints. He recommended conducting a systematic test to determine the practical design of prestressed concrete joints. Furthermore, a thorough examination of the factors of durability, anchorage rotation, kind of prestressing steel, and the ultimate strain needed for a prestressing system in a seismic zone is necessary[5].

To establish load-displacement hysteresis curves for fully and partially prestressed concrete joints under severed earthquake force, Thompson and Park conducted cyclic load tests. The test's findings show that prestressed beams' ductility was enhanced when non-prestressed reinforcing was present in the members' compression zones [6]. The load-displacement hysteresis curve was later adjusted by Nishiyama, who also examined the prestressed concrete structure under seismic tension [7]. In Japan, Nishiyama presented the prestressed concrete building seismic design process and contrasted it with traditional reinforced concrete constructions. According to the study, prestressing tendons in column-beam joints enhance the joint's ability to absorb energy and enhance its shear behaviour[8]. Priestley and Tao evaluate the earthquake resistance of precast prestressed concrete frames for tall buildings featuring partially deboned tendons[9].  The shear performance of the joint was found to be enhanced by the suggested approach, which only required nominal stirrups. According to the study, in order to ensure that the plastic hinges on the beam function properly, two percent of specific spiral reinforcement should be positioned in the area at the end of the beam. [9]. For constructions with partially deboned tendons, the ductility requirements would not be higher than those for fully bonded tendons in situations where prestress diminishes due to inelastic strains of the prestressing tendon, as several dynamic inelastic computations show.

An analytical and experimental investigation of the hysteresis restoring force characteristic of unbounded prestressed concrete frame structures subject to earthquake loading has been carried out by Nishiyama, Mugurama, and Watanabe. The analysis comes to the conclusion that unbounded prestressed concrete can be used in the earthquake scenario since the rotation of the beam ends is small, the tendon force is low, and the frame deformed in a significant inelastic range[10]. To evaluate the hysteresis characteristic of prestressed concrete frames subjected to variable loading histories, Su and Zhu developed a new analytical algorithm[11].  A study on the seismic behaviour and design of unbounded post-tensioned precast concrete frames was carried out by Kurama, Pessiki, Sause, and Lu. The study discovered that local ductility demand variables are necessary in order to raise the local ductility needs for the beam-column connections[12]. Due to the small energy dissipation, the maximum displacement of unbounded post-tensioned precast frames under seismic loading is expected to be higher than that of conventional cast-in-place reinforced concrete frames, while the cumulative residual displacement is expected to be much smaller. These local ductility requirement factors are higher for frames intended for high seismicity locations than for frames intended for moderate seismicity regions. By ysing the capacity spectrum method, Nishiyama has undertaken a study to anticipate how prestressed concrete buildings will respond to earthquake loading. The findings have indicated that prestressed concrete has a somewhat greater damping energy in comparison to ordinary reinforced concrete, and that its hysteresis energy is much greater than that of reinforced concrete. As a result, in the case of prestressed concrete, the seismic energy is dispersed kinematically[7].

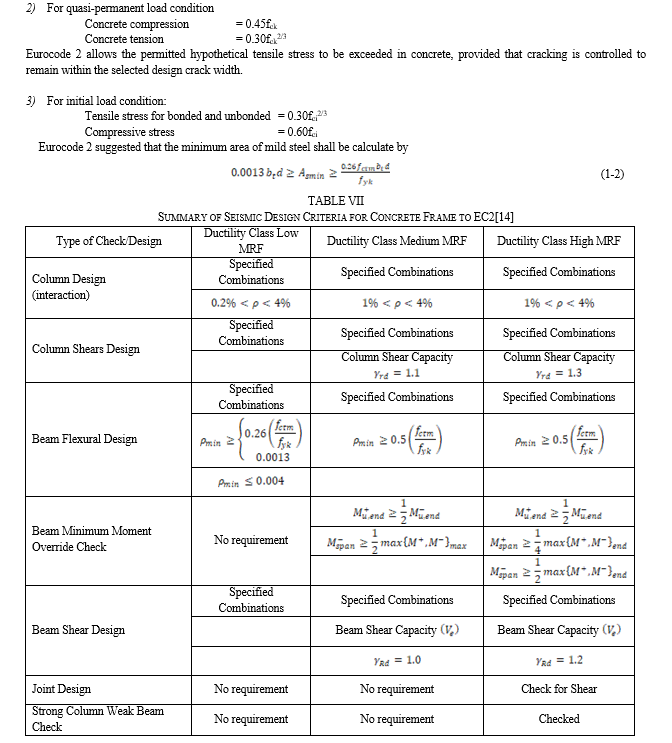
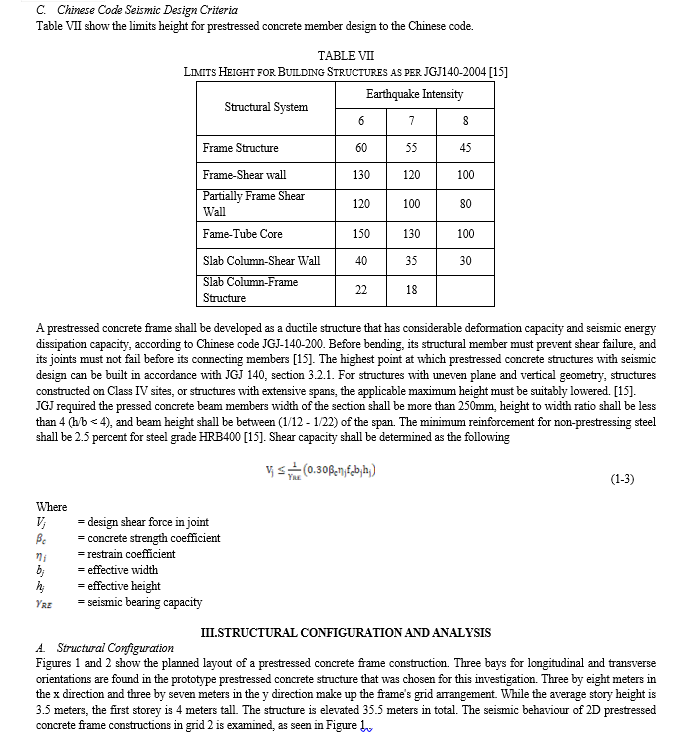
A. American Code Seismic Design Criteria

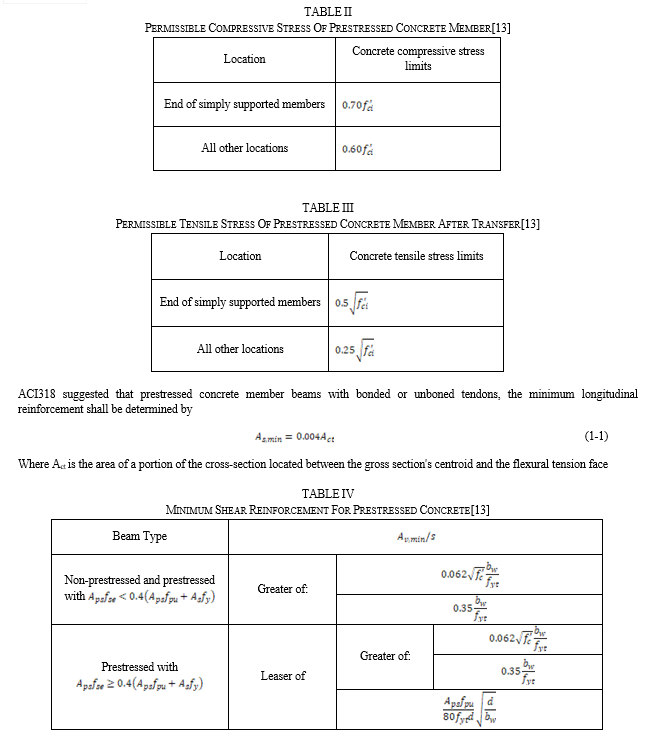
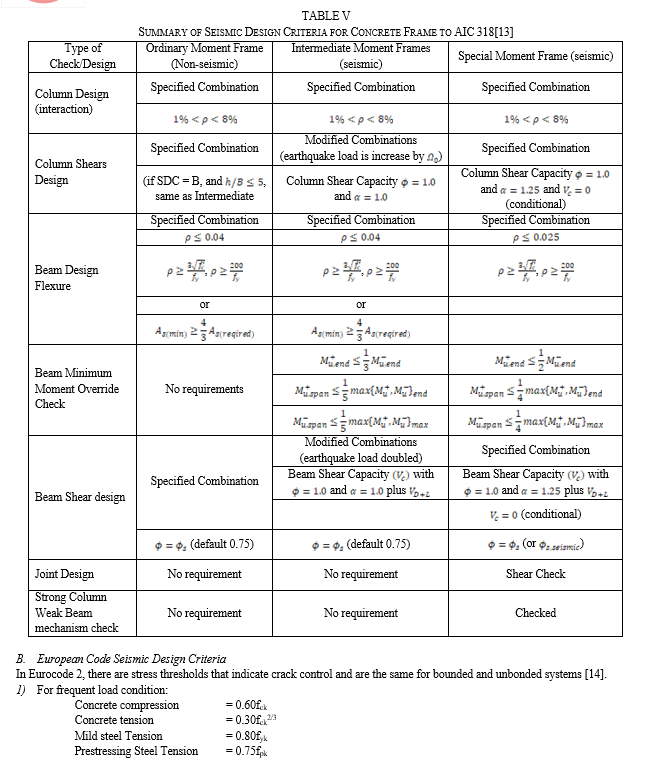
According to ACI318, there shall be three classes in the design of prestressed concrete members. Class T is the partially cracked member, Class U is the uncracked member, and Class C is the cracked member. Tensile stresses in the concrete should be reduced and the cover elevated in accordance with section 20.6.1.4 of ACI-318 to reduce the likelihood of cracking under service loads. ACI-318 suggests that prestressed concrete members be designed as gross sections for Class U and Class T to meet the serviceability criterion. In the interim, Class C will analyse using the cracked section. As per ACI-318, the following tables shall satisfy the permitted tensile and compressive stress in the prestressed concrete members. [13].

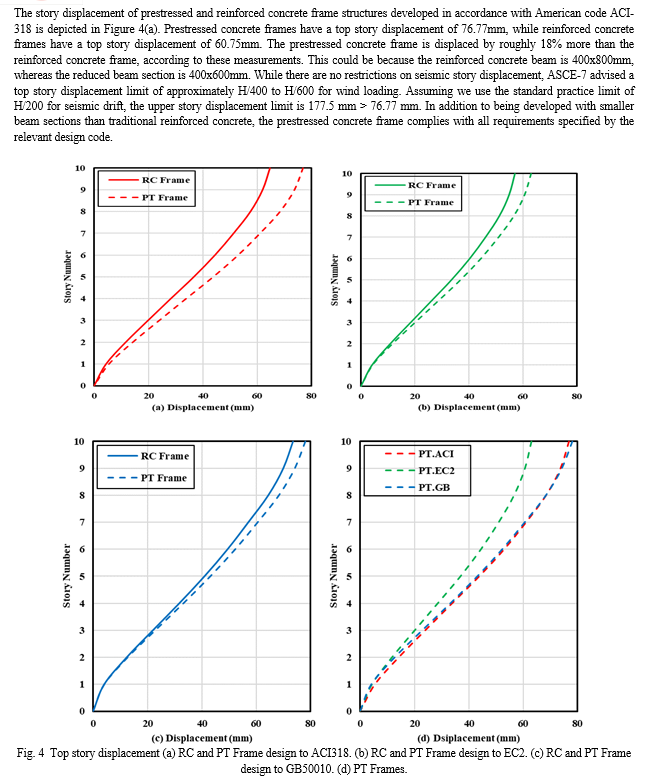
Reinforced and prestressed concrete frames for the top story replacement measure 57.14 mm and 62.95 mm, respectively. Because smaller beam sections are constructed into prestressed concrete frame structures, a prestressed concrete frame has a 10% higher displacement than a reinforced concrete frame. Nonetheless, the frame made of prestressed concrete remains within the permissible limits as stipulated by Eurocode 2 as shown in Figure 4(b).

The story displacement for prestressed and reinforced concrete frame structures developed in accordance with the Chinese code GB50010-2010 is depicted in Figure 4(c). Both constructions have about the same storey displacement. The top displacement of the reinforced frame is 73.52mm, whereas the prestressed concrete frame provides a displacement of 78mm. Precast and reinforced concrete frames are both permitted within the bounds set forth in GB50010-2010.

The top story displacement between prestressed concrete frames developed in accordance with GB50010-2010, Eurocode 2, and ACI-318 is depicted in Figure 4(d). The displacement of the prestressed concrete frame developed in accordance with ACI-318 and GB50010-2010 is nearly the same; both are roughly 20% greater than that of Eurocode 2. The material qualities and safety considerations listed in the relevant code are to blame for this outcome.

The inter-story drift of reinforced and prestressed concrete frame structures built in accordance with American code ACI-318 is depicted in Figure 5(a). As recommended by ASCE-7 provisioned, the aforementioned equation (3.10) is used to determine the inelastic drift. The reinforced concrete frame structure has a story drift of roughly 1.3%, while the largest story drift of the prestressed concrete frame structure is approximately 1.6% at story 5. Both of the reinforced concrete frame constructions meet the ASCE-7's acceptable standards.

The inter-story drift of the prestressed concrete frame structure, developed in accordance with the following Eurocode 2 regulation, is depicted in Figure 5(b). Table 13.4 is used to determine the inelastic drift. A 1.4% story drift is produced by the prestressed concrete frame and a 1.3% drift by the reinforced concrete frame. Regarding seismic drift, both systems are similar and meet Eurocode 8's acceptance requirements.

The inter-story drift of prestressed and reinforced concrete frame structures designed in accordance with Chinese code GB50010-2010 is depicted in Figure 5(c). The graphs demonstrate that while the drifts in the prestressed and reinforced concrete frame structures are nearly comparable, the prestressed concrete frame structure's drift is still more than the reinforced concrete's. Nevertheless, neither structure exceeds the GB50011 limit.

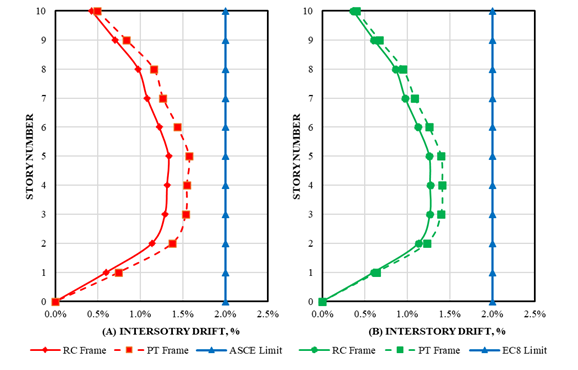
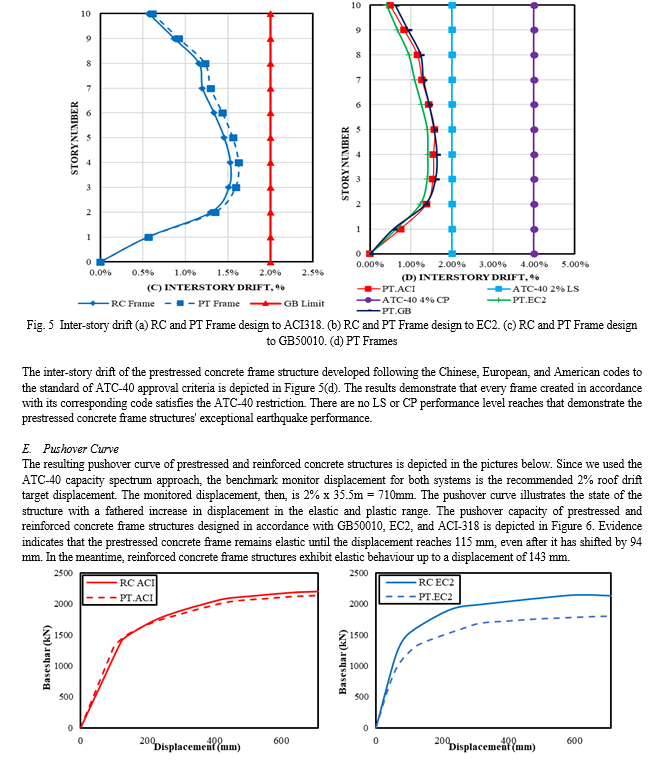
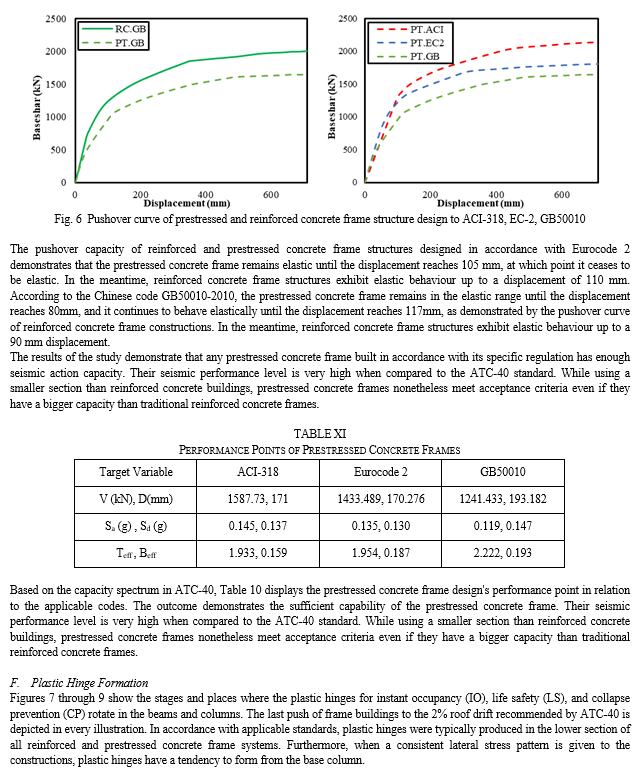
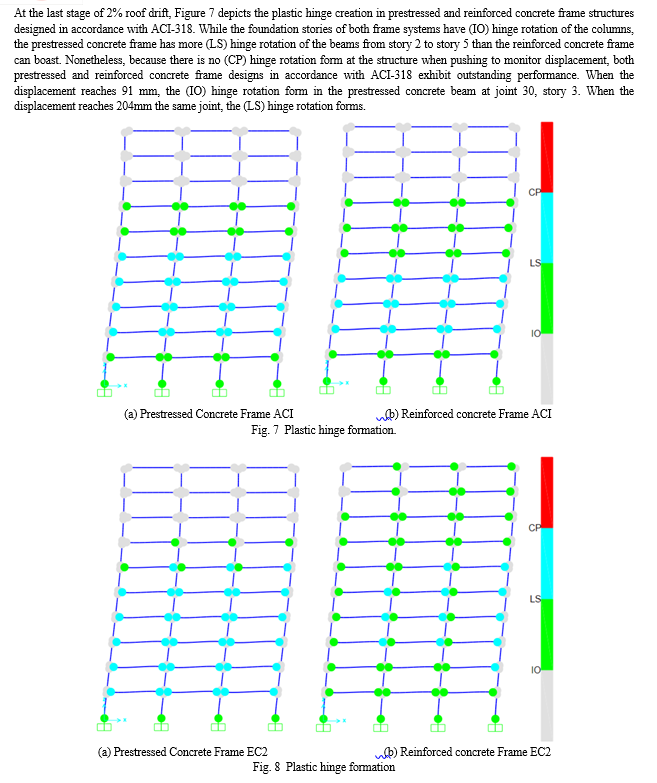
Figure 8 illustrates how prestressed and reinforced concrete frame constructions designed in accordance with Eurocode 2 rotate on plastic hinges. When the last push reach is displaced, both frame systems have (IO) hinge rotation in the columns at the base story; no (CP) hinge rotation form exists. Nonetheless, compared to reinforced concrete frame buildings, prestressed concrete frame structures have higher (LS) hinge rotation. Since there is no (CP) hinge rotation form in the columns or beams, both prestressed and reinforced concrete frames have demonstrated good performance levels.

The plastic hinge rotation of prestressed and reinforced concrete frame constructions designed in accordance with Chinese code GB50010-2010 is depicted in Figure 9. It is evident that prestressed concrete frame structures exhibit (LS) hinge rotation in the top part of the structure when it reaches the monitor displacement, but reinforced concrete frame constructions exhibit greater (LS) hinge rotation forms in their beams. As per the aforementioned pattern, neither the beams nor the columns for either system have any (CP) hinge rotation form.

V. ACKNOWLEDGMENT

I would like to thank my supervisor, Dr. Pan Zaunfeng, for his understanding and valuable time spent providing advice and direction for my stay at Tongji University.  He offered guidance and considerable time to help me accomplish my research and master's degree promptly.

Conclusion

The American, European, and Chinese codes for prestressed concrete frame structure design and their seismic behavior are the main topics of this study. Using the finite element program CSI Sap2000, the nonlinear static pushover process recommended by ATC-40, which is based on the capacity spectrum method, is used to analyze the seismic performance of the structures. The primary deductions drawn from the analytical outcome are: 1) The structure of the prestressed concrete frames is strong enough to withstand seismic activity while yet adhering to the damage restriction guidelines specified by the relevant design code. 2) The highest capacity is found in prestressed concrete frame designs according to ACI-318, which is followed by those according to Eurocode 2 and Chinese code GB50010, in that order. This is because each applicable code has a slight variation in the material attribute, safety factor, and loading assumption. 3) Though conventional reinforced concrete has a larger capacity, precast concrete frame structures maintain design satisfaction with smaller dimension properties. 4) The strong column weak beam mechanism is archived in the prestressed concrete frame structures when pushing to monitored displacement. 5) The 3 major design codes has a similar acceptance criteria to ATC-40 benchmark.

References

[1] W. M. Sutherland, “Prestressed Concrete Earthquake Resistant Structures-Development, Performance, and Current Research,” in Proceedings of 3rd World Conference on Earthquake Engineering, Vol. Ill, p. IV-463, 1965. [2] K. Nakano, Experiment on behaviour of prestressed concrete four storeyed model structure under lateral force. International Institute of Seismology and Earthquake Engineering, 196 [3] T. Y. Lin, “Design of prestressed concrete buildings for earthquake resistance,” Journal of the Structural Division, vol. 91, no. 5, pp. 1–17, 1965. [4] S. Inomata, “Comparative Study on behaviour of Prestressed and Reinforced Concrete Beams Subject to Loading Reversals,” PRECAST/PRESTRESSED CONCRETE INSTITUTE. JOURNAL, 1971. [5] N. M. Hawkins, “Seismic resistance of prestressed and precast concrete structures,” PCI JOURNAL, vol. 22, no. 6, pp. 80–110, 1977. [6] R. Park and K. J. Thompson, “Cyclic load tests on prestressed and partially prestressed beam-column joints,” PCI Journal, vol. 22, no. 5, pp. 84–110, 1977. [7] M. Nishiyama, “Prediction of Response of Prestressed Concrete Buildings to Earthquake Excitation Using Capacity Spectrum Method,” Journal of Advanced Concrete Technology, vol. 3, no. 2, pp. 225–231, 2005 [8] M. Nishiyama, “Seismic design of prestressed concrete buildings,” Bulletin of the New Zealand Society for Earthquake Engineering, vol. 23, no. 4, pp. 288–304, 1990. [9] M. J. N. Priestley and J. R. Tao, “Seismic response of precast prestressed concrete frames with partially debonded tendons,” PCI journal, vol. 38, no. 1, pp. 58–69, 1993. [10] M. Nishiyama, H. Mugurama, and F. Watanabe, “Hysteretic restoring force characteristics of unbounded prestressed concrete framed structure under earthquake load,” Bulletin of the New Zealand Society for Earthquake Engineering, vol. 22, no. 2, pp. 112–121, 1989. [11] X. Su and B. Zhu, “Algorithm for hysteresis analysis of prestressed-concrete frames,” Journal of Structural Engineering, vol. 120, no. 6, pp. 1732–1744, 1994. [12] B. D. Weldon and Y. C. Kurama, “Experimental evaluation of posttensioned precast concrete coupling beams,” Journal of Structural Engineering, vol. 136, no. 9, pp. 1066–1077, 2010. [13] ACI Committee 318, “BUILDING CODE REQUIREMENTS FOR STRUCTURAL CONCRETE (ACI 318-14) AND COMMENTARY (ACI 318R-14),” Sep. 2014. [14] B. Standard, Eurocode 2: Design of concrete structures, vol. 1, no. 1. 2004. [15] JGJ 140, “Specification for seismic design of prestressed concrete structure,” 2004 [16] American Society of Civil Engineers, Minimum Design Loads and Associated Criteria for Buildings and Other Structures. Reston, VA: American Society of Civil Engineers, 2017. doi: 10.1061/9780784414248. [17] B. Standard, Eurocode 1: Actions on structures, vol. 196. 2006. [18] GB50009, Load code for the design of building structures. GB National Standard, 2012. [19] W. Y. Luo Kaihai, “Research on conversion relationships among the parameters of ground motions seismic design codes of China, America and Europe,” Building Structure, vol. 36, no. 8, pp. 103–107, 2006. [20] A. J. Pierre and I. Hidayat, “Seismic performance of reinforced concrete structures with pushover analysis,” in IOP Conference Series: Earth and Environmental Science, 2020, p. 12045. [21] ATC-40, “Seismic Evaluation and Retrofit of Concrete Buildings,” Redwood City, Nov. 1996.

Copyright

Copyright © 2024 Ratanak Mony. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.